Decibel & S-Readings
Serge Stroobandt, ON4AA
Copyright 2015–2021, licensed under Creative Commons BY-NC-SA
Base-10 logarithms: log10 x
\(\log_{10}{x}=\dots\quad\) means: “To what power do I need to raise 10, in order to obtain x?”
\[\log_{10}{x}=y\quad\Leftrightarrow\quad 10^y=x\]
dB as a power ratio
The decibel (dB) is a logarithmic unit used to express the ratio of two values of a physical quantity.1 For power ratios the decibel unit is defined as follows:
\[L_{dB}=10\cdot\log_{10}{\frac{P_{out}}{P_{in}}}\]
dB as a field amplitude ratio
For intensity ratios the decibel unit is defined as follows:
\[G_{dB}=20\cdot\log_{10}{\frac{A_{out}}{A_{in}}}\]
Decibel conversion table
| dB | \(\frac{P_{out}}{P_{in}}\) | \(\frac{A_{out}}{A_{in}}\) |
|---|---|---|
| 40 | 10000 | 100 |
| 30 | 1000 | ≈31.62 |
| 20 | 100 | 10 |
| 10 | 10 | ≈3.162 |
| 6 | ≈ 4 | ≈ 2 |
| 3 | ≈ 2 | ≈ \(\sqrt{2}\) ≈ 1.414 |
| 1 | ≈ 1.25 | ≈ 1.125 |
| 0 | 1 | 1 |
| -1 | ≈0.8 | ≈0.9 |
| -3 | ≈ \(\frac{1}{2}\) = 0.5 | ≈ \(\frac{1}{\sqrt{2}}\) ≈ 0.707 |
| -6 | ≈ \(\frac{1}{4}\) = 0.25 | ≈ \(\frac{1}{2}\) = 0.5 |
| -10 | 0.1 | ≈0.3162 |
| -20 | 0.01 | 0.1 |
| -30 | 0.001 | ≈0.03162 |
| -40 | 0.0001 | 0.01 |
dBm as a power level
dBm is a logarithmic unit of power level, expressed in decibel (dB) and referenced to a power level of one milliwatt (mW).2
| dBm | \(P_{out}\) | typical for |
|---|---|---|
| 60 | 1kW | typical radiated RF power of a microwave oven |
| 50 | 100W | typical maximum output RF power from a ham radio HF transceiver |
| 40 | 10W | |
| 37 | ≈ 5W | typical maximum output RF power from a handheld ham radio VHF/UHF transceiver |
| 33 | ≈ 2W | maximum output from a GSM 850/900 mobile phone |
| 30 | 1W | DCS or GSM 1 800/1 900 MHz mobile phone |
| 20 | 100mW | EIRP for a IEEE 802.11b/g 20 MHz-wide channel in the 2.4 GHz ISM band (5 mW/MHz) |
| 10 | 10mW | |
| 0 | 1mW | Bluetooth class 3 radio with 1 m range |
| -10 | 100µW | IEEE 802.11 maximal signal strength |
| -60 | 1nW | power received per m2 of a magnitude +3.5 star |
| -73 | ≈ 50pW | S9 signal strength on S-meter |
| -100 | 100fW | IEEE 802.11b/g minimal signal strength |
| -101 | ≈ 83fW | noise floor of a IEEE 802.11b/g 20 MHz channel at 300 K |
| -134 | ≈ 41aW | noise floor of a 10 kHz wide FM signal at 300 K |
| -140 | ≈ 12aW | noise floor of a 2.7 kHz wide SSB signal at 300 K |
In this table, the term noise floor refers to the calculated thermal noise, also known as the Johnson–Nyquist noise.3
HF S-meter
Many amateur radio and shortwave broadcast receivers feature a signal strength meter (S‑meter).4 In 1981, the International Amateur Radio Union (IARU) Region 1 agreed on a technical recommendation for S‑meter calibration of HF and VHF/UHF transceivers.5,6
IARU Region 1 Technical Recommendation R.1 defines S9 for the HF bands to be a receiver input power of -73 dBm. This is a level of 50 µV at the receiver’s antenna input assuming the input impedance of the receiver is 50 Ω.
The recommendation defines a difference of one S-unit corresponds to a difference of 6 dB, equivalent to a voltage ratio of two, or a power ratio of four. Signals stronger than S9 are given with an additional dB rating, thus “S9 + 20 dB”, or, verbally, “20 decibel over S9”, or simply “20 over 9” or even the simpler “20 over.”
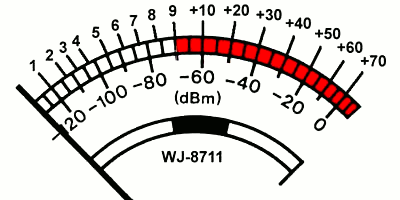
Well-designed S-meter on the DRS WJ-8711A HF transceiver. Source: N9EWO
| S-reading | \(P_{out}\) @50Ω | \(V_{out}\) @50Ω | \(\frac{V_{out}}{\left[1\,\text{µV}\right]}\) @50Ω |
|---|---|---|---|
| S9 + 40 dB | -33 dBm | 5.0 mV | 74 dBµV |
| S9 + 30 dB | -43 dBm | 1.6 mV | 64 dBµV |
| S9 + 20 dB | -53 dBm | 0.50 mV | 54 dBµV |
| S9 + 10 dB | -63 dBm | 0.16 mV | 44 dBµV |
| S9 | -73 dBm | 50 µV | 34 dBµV |
| S8 | -79 dBm | 25 µV | 28 dBµV |
| S7 | -85 dBm | 12.6 µV | 22 dBµV |
| S6 | -91 dBm | 6.3 µV | 16 dBµV |
| S5 | -97 dBm | 3.2 µV | 10 dBµV |
| S4 | -103 dBm | 1.6 µV | 4 dBµV |
| S3 | -109 dBm | 800 nV | -2 dBµV |
| S2 | -115 dBm | 400 nV | -8 dBµV |
| S1 | -121 dBm | 200 nV | -14 dBµV |
The noise floor for a \(B=2700\) Hz wide SSB signal at \(T=300\) K is:3
\(P=k_B\cdot T\cdot B=k_B\cdot300\cdot2700=11.8\cdot10^{-18}\,\text{W}=11.8\text{aW}=-139.5\,\text{dBm}\)
where \(k_B=1.3806488\cdot10^{-23}\,\text{J/K}\) is Boltzmann’s constant.
VHF/UHF S-meter
The same IARU Region 1 recommendation defines S9 for VHF/UHF to be a receiver input power of -93 dBm. This is the equivalent of 5 µV in 50 Ω. Again, one S-unit corresponds to a difference of 6 dB, equivalent to a voltage ratio of two, or a power ratio of four.
| S-reading | \(P_{out}\) @50Ω | \(V_{out}\) @50Ω | \(\frac{V_{out}}{\left[1\,\text{µV}\right]}\) @50Ω |
|---|---|---|---|
| S9 + 40 dB | -53 dBm | 0.50 mV | 54 dBµV |
| S9 + 30 dB | -63 dBm | 0.16 mV | 44 dBµV |
| S9 + 20 dB | -73 dBm | 50 µV | 34 dBµV |
| S9 + 10 dB | -83 dBm | 16 µV | 24 dBµV |
| S9 | -93 dBm | 5.0 µV | 14 dBµV |
| S8 | -99 dBm | 2.5 µV | 8 dBµV |
| S7 | -105 dBm | 1.26 µV | 2 dBµV |
| S6 | -111 dBm | 630 nV | -4 dBµV |
| S5 | -117 dBm | 320 nV | -10 dBµV |
| S4 | -123 dBm | 160 nV | -16 dBµV |
| S3 | -129 dBm | 80 nV | -22 dBµV |
| S2 | -135 dBm | 40 nV | -28 dBµV |
| S1 | -141 dBm | 20 nV | -34 dBµV |
The noise floor for a 10 kHz wide FM signal at 300 K is:3
\(P=k_B\cdot T\cdot B=k_B\cdot300\cdot10^{4}=41\cdot10^{-18}\,\text{W}=41\text{aW}=-134\,\text{dBm}\)
where \(k_B=1.3806488\cdot10^{-23}\,\text{J/K}\) is Boltzmann’s constant.
References

This work is licensed under a Creative Commons Attribution‑NonCommercial‑ShareAlike 4.0 International License.
Other licensing available on request.

Unless otherwise stated, all originally authored software on this site is licensed under the terms of GNU GPL version 3.
This static web site has no backend database.
Hence, no personal data is collected and GDPR compliance is met.
Moreover, this domain does not set any first party cookies.
All Google ads shown on this web site are, irrespective of your location,
restricted in data processing to meet compliance with the CCPA and GDPR.
However, Google AdSense may set third party cookies for traffic analysis and
use JavaScript to obtain a unique set of browser data.
Your browser can be configured to block third party cookies.
Furthermore, installing an ad blocker like EFF's Privacy Badger
will block the JavaScript of ads.
Google's ad policies can be found here.
transcoded by
 .
.


